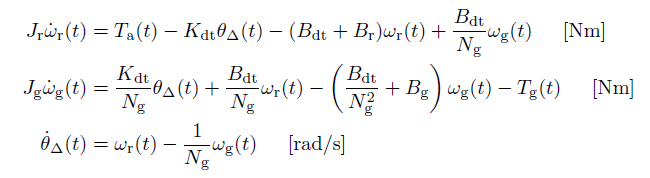Dear All,
In the following equations:
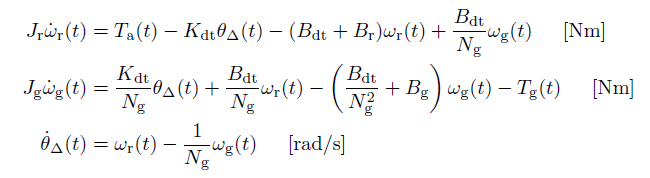
What are the values of the following parameters for the 5-MW WT?
The torsion damping coefficient of the drive train (Bdt) [Nm/(rad/s)]?
The torsion stiffness of the drive train (Kdt) [Nm/rad]?
The viscous friction of the high-speed shaft (Bg) [Nm/(rad/s)]?
The viscous friction of the low-speed shaft (Br) [Nm/(rad/s)]?
(State variable “theta” is the torsion angle of the drive train)
Dear Sina,
You can use the linearzation functionality of FAST / OpenFAST to derive the coefficients of a linear model. It looks like your model involves two degrees of freedom (DOF)–the generator and drivetrain-torsion DOF. So using the NREL 5-MW baseline turbine FAST model, enable these DOFs, identify your operating point (wind speed / rotor speed / pitch angle), and linearize.
Best regards,
Dear Dr. Jonkman,
Thanks for your reply that is as always with celerity. I am using Fast 8, my question is that whether I need to switch to Fast 7 for linearization or not to attain those coefficients?
Best regards,
Sina
Dear Sina,
If you divide the first equation by J_r and the second equation by J_g, you can get the resulting coefficients out of the linearization capability of FAST v8. The linearization capability of FAST v8 will not provide the mass matrix directly (although you can infer it by looking the Jacobians of the full module inputs and outputs).
Best regards,
Dear Dr. Jonkman,
Thanks again for your reply. As I understood, since Fast 8 gives a Jacobians matrix, if I divide the equations by those moments, I can calculate each coefficient using those moments. How can I get the Jacobians matrix using Fast 8? Is this matrix generated in Matlab? What is the procedure of generating such a matrix using Fast 8?
Best regards,
Sina
Dear Sina,
The linearization process is used to calculate and return the matrices of a linear state-space model representing the state and output equations about the operating point. The linear matrices of this state-space model are the Jacobians. See our TORQUE 2016 paper for more information on the theoretical basis of the linearization process: wind.nrel.gov/nwtc/docs/README_FAST8.pdf.
Best regards,
Dear Dr. Jonkman,
Thank you so much for clarifying the issue and introducing the references.
Best regards,
Sina
Dear Dr. Jonkman,
I have two questions about the 5MW wind turbine modeled by FAST.
First, does FAST modeled torsion angle that is located in the formula I put above? I realized that in FAST (test 18) Wg=Ng*Wr assuming that there is no speed change in shafts. (It seems it only considers the steady state but not transient state, i.e, there is no dynamic for it!) Is there any other test considering this effect?
Second, you mentioned in your previous comments that I need to linearize the model to obtain those coefficients. Are they dependent on various operating points?
Thanks
Best Regards,
Sina
Dear Sina,
I’m not sure I understand your first question, but FAST and Test18 do include the drivetrain-torsion degree of freedom (DOF). The generator speed is proportional to the low-speed shaft speed at the entrance to the gearbox (via the gearbox ratio), but there is a torsional DOF (with spring-damper) in the low-speed shaft between the gearbox and rotor.
Yes, the coefficients of the linear model will certainly depend on the operating point (because many parts of the FAST model are inherently nonlinear).
Best regards,
Dear Dr. Jonkman,
Thanks for your prompt reply. Regarding my first question, yes, having it simulated, the transient response Wg was not equal to Ngear times Wr but for the steady state response generator speed is equal to gear ratio times rotor speed as it is in the simulation. My second question was about the viscous friction and torsion damping coefficient and torsion stiffness (not the state space matrix that should be obtained from the linearization technique around equilibrium points).
Thanks
Best regards,
Sina
Dear Sina,
The structural stiffness and damping related to the drivetrain torsion are linear (and so, are independent of the operating point), but the aerodynamic stiffness and damping are likely dependent on the operating point.
Best regards,
Dear Dr. Jonkman,
Thank you so much for clarifying the issue.
Best regards,
Sina